

Curve Balls Sweet Spot / Corked Bats / Questec-MLB Zone Evaluation
A ball that would travel 400 feet in "normal" conditions goes:
6 feet farther if the altitude is 1,000 feet higher
4 feet farther if the air is 10 degrees warmer
4 feet farther if the ball is 10 degrees warmer
4 feet farther if the barometer drops 1 inch of mercury
3 1/2 feet farther if the pitcher is 5 mph faster
30 feet farther if struck with an aluminum bat
To hit a ball the maximum possible distance, the trajectory off the bat should have a 35-degree angle.
A line drive travels 100 yards in 4 seconds. A fly to the outfield travels 98 yards in 4.3 seconds.
An average head wind (10 mph) can turn a 400-foot home run into a 370-foot routine out.
A curveball that seems to break over 14 inches never actually deviates from a straight line more than 3 1/2 inches.
Part of the ball's deviation from a straight line is governed by the equation:
[delta]P=PR-PL=1/2 Þair [vL2 - vR2 ]
which describes the magnitude of the pressure differential between the left and right sides of a rotating, thrown baseball.
here is no possible way (excluding softball) to throw a rising fastball that actually rises.
Excluding meteorologically strange conditions, a batted ball cannot travel longer than 545 feet.
The collision of a bat and baseball lasts only approximately 1/1000 of a second.
Good news for batters: The "muzzle velocity" of a pitched baseball slows down about 1 mph every 7 feet after it leaves the pitcher's hand, that's a loss of roughly 8 mph by the time it crosses the plate.
Bad news for batters: If you swing 1/100th of a second too soon a ball will go foul down the left field side (right handed batter). 1/100th of a second too late and it's foul in the right field seats, and the decision to swing has to happen within 4/100th of a second.
For over a century baseball fans have debated the question of whether a "curve ball does in fact curve". Only rarely has there been objective scientific testing in order to verify what is so obviously the appearance of a curve.
Igor Sikorsky's interest had stemmed from a phone call he received from United Aircraft's Lauren (Deac) Lyman who over lunch with Walter Neff of United Airlines, had discussed the question of the trajectory of a baseball.
Mr. Sikorsky, who has a wind tunnel, called his engineers together presenting the problem as follows: "Here we have a solid sphere, moving rapidly in space and rotating on a vertical axis. You see? ... the object is to elude the man with the stick". It should be noted that baseball was a rather foreign endeavor to Mr. Sikorsky.
Being a man of
science he realized that a pitched ball, traveling in a curved path, is an example of aerodynamic action in everyday life. This force which causes a spinning ball to curve in flight is the "Magnus effect".

Sikorsky's first problem was to determine how much spin a pitcher could put on a baseball in the regulation sixty-foot, six-inch distance from the mound to the plate. Engineers who were baseball fans were glad to contribute some of their off-duty time. Careful studies were made of fast-motion photographs showing the process of a single pitch. Studying the change in the position of the baseball's stitches from picture to picture proved that the rate of rotation was about five revolutions for the pitch, or about 600 revolutions per minute.
The next problem was to determine if this spin could cause a baseball to curve in flight. Testing began in the Sikorsky Vertical Wind Tunnel during the next "stand-by time" between aircraft model performance tests.
Since Big Leaguer's fast balls were officially clocked at 98.6 miles per hour, the forward speeds of the air moving through the wind tunnel was varied between 80 and 110 miles per hour.
Using official National and American League baseballs - identical except for their markings - Sikorsky impaled them on a slender spike connected to the shaft of a small motor and rotated them between zero and 1,200 revolutions per minute. The motor was mounted on a delicately-balanced scale which measured the direction and force of all pressure brought on the baseballs.
To observe maximum and minimum effects the baseballs were spiked and rotated at two different angles. In one position four seams met the wind during each revolution. This they found produced the greatest amount of side force. Only two seams met the wind in the other test position causing less friction and less side force.
Sikorsky's conclusions were that the baseball will in fact curve in the sense that the spinning baseball does follows a steady arc, rather than traveling in a straight line and then "Breaking". A pitcher who can release the baseball so that four seams meet the wind can "Break" as much as 19 inches. With the same speed and rotation a two seam pitch will break 7.5 inches.
To the batter, who views the baseballs flight at an angle, it appears that the baseball travels fairly straight most of the way and then "Breaks" suddenly and sharply near the plate, this is an optical illusion.
Note: Perception plays a big role in the curve ball: The typical curveball goes through only 3.4 inches of deviation from a straight line drawn between the pitcher’s hand and the catcher’s glove. However, from the perspective of the pitcher and batter, the ball moves 14.4 inches. This proves that a curve ball really curves. The wind is also a major factor in perception of the total break.
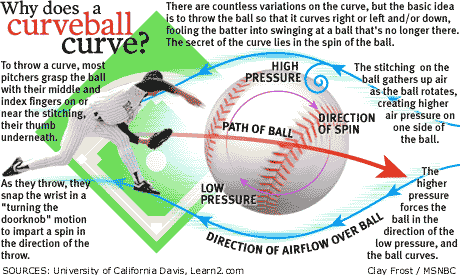
The secret to understanding a curveball is the speed of the air moving past the ball's surface. A curve has topspin, meaning that the top of the ball is moving in the same direction as the throw and the OPPOSITE direction of air flow relative to the direction of the throw. Vice versa for the bottom of the ball. It moves in the SAME direction as the air flow relative to the throw. See Bernoulli's principle, which says that the lower velocity of the air over the ball creates more pressure on the ball, which is what makes the curveball break downward. (Thanks to Lizbeth for correcting this info))
What difference does that make? The higher velocity difference puts more stress on the air flowing around the bottom of the ball. That stress makes air flowing around the ball "break away" from the ball's surface sooner. Conversely, the air at the top of the spinning ball, subject to less stress due to the lower velocity difference, can "hang onto" the ball's surface longer before breaking away.
As a result, the air flowing over the top of the ball leaves it in a direction pointed a little bit downward rather than straight back. As Newton discovered almost three hundred years ago, for every action there is an equal and opposite reaction. So, as the spinning ball throws the air down, the air pushes the ball up in response. A ball thrown with backspin will therefore get a little bit of lift.
A major league curveball can veer as much as 171/2 inches from a straight line by the time it crosses the plate. Over the course of a pitch, the deflection from a straight line increases with distance from the pitcher. So curveballs do most of their curving in the last quarter of their trip. Considering that it takes less time for the ball to travel those last 15 feet (about 1/6 of a second) than it takes for the batter to swing the bat (about 1/5 of a second), hitters must begin their swings before the ball has started to show much curve. No wonder curveballs are so hard to hit.
One important difference between a fastball, a curveball, a slider, and a screwball is the direction in which the ball spins. (Other important factors are the speed of the pitch and rate of spin.) Generally speaking, a ball thrown with a spin will curve in the same direction that the front of the ball (home plate side, when pitched) turns. If the ball is spinning from top to bottom (topspin), it will tend to nosedive into the dirt. If it's spinning from left to right, the pitch will break toward third base. The faster the rate of spin, the more the ball's path curves.
 A baseball bat has three "sweet spots"; one of them is called its "center of percussion" (COP). That's physicist talk for the point where the ball's impact causes the smallest shock to your hands. If you hit a baseball closer to the bat's handle than to the center of percussion, you'll feel a slight force pushing the handle back into the palm of your top hand. If you hit the ball farther out than the COP, you'll feel a slight push on your fingers in the opposite direction, trying to open up your grip. But if you hit the ball right on the COP, you won't feel any force on the handle. To find the COP on a bat, try this simple activity.
A baseball bat has three "sweet spots"; one of them is called its "center of percussion" (COP). That's physicist talk for the point where the ball's impact causes the smallest shock to your hands. If you hit a baseball closer to the bat's handle than to the center of percussion, you'll feel a slight force pushing the handle back into the palm of your top hand. If you hit the ball farther out than the COP, you'll feel a slight push on your fingers in the opposite direction, trying to open up your grip. But if you hit the ball right on the COP, you won't feel any force on the handle. To find the COP on a bat, try this simple activity.
Requirements:
A bat
A ball
A friend
What To Do and Look For:
When you hold a bat with your hands at the bottom of the handle (a normal grip), the COP is located about six to eight inches from the fat end of the bat. If you choke up on the bat, the COP moves closer to the fat end. That's because the location of your top hand is the place you want the bat to pivot. Changing your hand's position on the bat changes where that pivot point is, which therefore changes the position of the COP to one that corresponds to the new pivot point.
To find the COP on a bat, hold it parallel to the ground in your hand. Make sure you hold it at the same place you normally do when playing a game. It's easier to feel the push if you hold the bat with only one hand; a two-handed grip helps to counteract the push in either direction. But be sure to hold it with the top hand in its "normal" position, no closer to the handle knob than you normally put your top hand. Close your eyes, so you can concentrate on the sensations you feel with your hand.
Have a friend throw a ball at the bat from a few inches away, starting at the end farthest from your hand and moving down the bat. The harder he or she can throw it, the better (as long as they're able to control where on the bat they're throwing the ball). Notice how the bat feels in your hand as the ball hits it. When we tried this at the Exploratorium, we could feel both a vibration and a force pushing on our hands. The amount of vibration and "push" varied, depending on where on the bat the ball hit. Some of us found it a little hard to distinguish between the two feelings, but if you can, the COP is where you feel the smallest push on your hand.
What's Happening?
A bat is essentially a long stick. When you hit a stick off center, two things happen: The entire stick wants to move straight backward, and it also wants to rotate around its center. It's this tendency to rotate that makes the bat's handle push back on or pull out of your hands.
When the ball hits the bat's COP, you don't feel a push or pull as the bat tries to spin. That's because when the bat spins, it pivots around one stationary point. When you hit a ball at the COP, the stationary point coincides with where your top hand is. So your hand feels no push one way or the other.
This is important if you want to hit the ball a long way. Every time you hit a ball at a point that's not the COP of your bat, some of the energy of your swing goes into moving the bat in your hands, not to pushing the ball so that it moves away from you farther and faster. If less of the bat's energy goes to your hands, more of it can be given to the ball.
The natural frequency of wooden bats is around 250 cycles per second, or 250 Hertz. Because the ball leaves the bat so soon (1 millisecond), the energy transfer to the ball is not too efficient. If the bat has been hollowed and corked, it's no longer as stiff and it will get an even lower natural frequency and an even less efficient transfer of energy to the bat. The baseball bounces off the bat faster than the cork can store the energy that could be put back in the ball. The cork might deaden the sound of a hollowed out bat, but it doesn't propel the ball. It can't. So, balls hit with corked bats don't go as far.
Some Remarks on Corked Bats
Alan M. Nathan
A corked bat is one in which a cavity has been drilled axially into the barrel of a wood bat. Typically, the diameter of the cavity is approximately 1 inch and it is drilled to a depth of about 10 inches. The cavity may or may not be filled with some substance, such as compressed cork, small superballs, etc.
Because wood has been removed from the bat and (possibly) replaced by some substance with a smaller density than wood, the bat is lighter by 1-2 oz., depending on the dimensions of the cavity and the density of the filling substance. Not only is the bat lighter, but the center of gravity, or balance point, of the bat moves closer to the hands. This means that the “swing weight” of the bat is also reduced. In technical physics language, the moment of inertia (MOI) of the bat about the knob is reduced for a corked bat. You can think of the MOI as the "rotational inertia" of the bat. Just like the "inertia" or mass of an object measures the resistance of the object to a change in its translational motion, the rotational inertia measures the resistance to a change in its rotational motion. The effect is easy to understand: It is much easier to swing something when the weight is concentrated closer to your hands (smaller MOI) than when it is concentrated far from your hands (larger MOI). You can try such an experiment yourself. Simply take a bat by the handle and swing try to rotate it rapidly. Then turn the bat around, holding the barrel, and try doing the same thing. You should find that it is easier to rotate it in the second case. Therefore, a batter can often get a higher bat speed with a corked bat than with a comparable bat that has not been corked. All other things being equal, a higher swing speed gives rise to a higher hit ball speed and greater distance on a long fly ball. Of course, all other things are not equal, and the reduced mass in the barrel produces a less effective collision, as we shall see in the next section.
An additional effect is that the lighter weight and smaller swing weight also lead to better bat control, which has a beneficial effect for a contact-type hitter, who is just trying to meet the ball squarely rather than get the highest batted ball speed. The batter can accelerate the bat to high speed more quickly with a corked bat, allowing the batter to react to the pitch more quickly, wait longer before committing on the swing, and more easily change in mid-swing. As has been pointed out by Bob Adair in his book, a batter can achieve the same effect legally by choking up on the bat or by using a lighter (and therefore probably shorter) bat. Of course, there are reasons one might not want to either choke up or use a shorter bat, especially in situations where you need to protect the outside part of the plate. In such a situation, a corked bat can provide a definite advantage. Many fast-pitch softball players take the issue of bat control to the extreme.
The efficiency of the bat in transferring energy to the ball in part depends on the weight of the part of the bat near the impact point of the ball. For a given bat speed, a heavier bat will produce a higher hit ball speed than a lighter bat. That is why the head of a golf driver is heavier than that of an iron: you want to drive the ball further. By reducing the weight at the barrel end of the bat, the efficiency of the bat is reduced, giving rise to a reduced hit ball speed and less distance on a long fly ball. This is the downside of using a corked bat.
We see that corking the bat leads to higher swing speed but to a less efficient ball-bat collision. These two effects roughly cancel each other out, leaving little or no effect on the hit ball speed or on the distance of a long fly ball. A specific example showing how this happens will be given below.
The trampoline effect is quite well known in hollow metal bats. The thin metal shell actually compresses during the collision with the ball and springs back, much like a trampoline, resulting in much less loss of energy (and therefore a higher batted ball speed) than would be the case if the ball hit a completely rigid surface. The loss of energy that I referred to comes mostly from the ball. During the collision, the ball compresses much like a spring. The initial energy of motion (kinetic energy) gets converted to compressional energy (potential energy) that is stored up in the spring. The spring then expands back out again, pushing against the bat, and converting the compressional energy back into kinetic energy. This is a very inefficient process in that only about 25% of the stored compressional energy is returned to the ball in the form of kinetic energy. The rest is lost due to frictional forces, deformation of the ball, etc. You can see the effect of this energy loss for yourself. Drop a baseball onto a hard rigid surface, such as a solid wood floor. The ball bounces back up to only a small fraction of its initial height because energy was lost in the collision of the ball with the floor. The loss mainly came from compressing and then expanding the ball. When a ball collides with a flexible surface, like the thin wall of an aluminum bat, the ball compresses less than it does when colliding with a rigid surface, since the thin wall does some of the compressing instead. Less energy is stored and ultimately lost in the ball, whereas the flexible surface is very efficient at returning its compressional energy back to the ball in the form of kinetic energy. The net effect is that the ball bounces off the flexible surface with higher speed than it does off the rigid surface. This is the essence of the trampoline effect. By the way, the trampoline effect is well known to tennis players, where the effect comes from the strings of the racket. All tennis players know that to hit the ball harder, you should decrease rather than increase the tension in the strings. Many people who do not play tennis find this counterintuitive, but it really is true. The lower tension makes the strings more flexible, just like a trampoline. You can even try the following experiment. Drop a baseball from the floor and measure the ratio of final height to initial height. Now drop a baseball from the strings of a tennis racket, making sure that the frame of the racket is clamped down so it does not vibrate. You should find that the ratio of final to initial height is higher than when the ball is dropped onto the floor. That is the trampoline effect in action.
With that long introduction, we come back to our question: Is there a trampoline effect from the hollowed-out wood bat or the cork filler? My own understanding of the physics of the ball-bat collision suggests that the answer is “no”. Why not? A 1”-diameter hole in a 2-1/2” diameter wood bat means the wall thickness is ¾”, which is at least 7 times thicker than that of a typical aluminum bat. It requires much greater force to compress such a bat than it does to compress an aluminum bat. In the technical parlance of physics, the spring constant of the hollow wood bat is much larger than that of a typical aluminum bat. Therefore, very little compressional energy is stored in the hollow wood bat during the collision, so that any trampoline effect is minimal at best.
In order to test this idea, I did an experiment several years ago with Professor Jim Sherwood at the Baseball Research Center (which Jim directs) at the University of Massachusetts/Lowell. We took two identical Louisville Slugger R161 wood bats, each with a length of 34” and a weight of 32.5 oz. Into one bat I drilled a 7/8 diameter hole, 9-1/4 deep into the barrel, removing a total of 2.0 oz. of wood. We then measured the ball exit speed when a 70 mph ball impacted the bat at a point 6 from the end of the bat. The speed of the bat at that point was set at 66 mph. Using the measured exit speed, the known inertial properties of the bats, and appropriate kinematic formulas, we extracted the ball-bat coefficient of restitution (COR), which is a measure of the liveliness of the ball-bat combination. We found the COR to be identical for the two bats, at least within the overall precision of the experiment. Had there been a trampoline effect, one would have found a larger COR for the hollowed bat. Armed with this information, I then did a calculation of hit ball speed that one would expect in the field, assuming a pitch speed of 90 mph and a bat speed that was slightly higher for the hollowed bat, based on a model for the relationship between bat swing speed and the swing weight of the bat. The model is based on the (unpublished) experimental study of Crisco and Greenwald, which gives a definite relationship between the MOI of the bat and the swing speed. The calculation shows that the unmodified bat actually performs slightly better than the hollowed bat (see figure below).
|
Figure 1. Calculation of hit ball speed from two otherwise identical wood bats. Relative to the normal bat, the corked bat had a cavity in the barrel of diameter 0.875” and depth 9.25”, thereby removing a total mass of 2 oz. from the barrel of the bat. The calculation assumes that the ball-bat COR is the same for each bat, as shown from experiment, and assumes a particular relationship between the bat swing speed and the moment of inertia of the bat. The calculation shows that the normal bat slightly outperforms the corked bat.
This is an interesting question. A more generic question is whether there is some substance that is compressible (so as to store energy) but not so compressible that it does not return the energy to the ball. This is a question that is worth thinking hard about and worth doing some experimental measurements to study the effect. Such experiments are currently in the planning stage.
And the Bottom Line?
It is quite unlikely that corking the bat will produce any appreciable effect, either of a beneficial or a detrimental nature, on the distance of a long fly ball. It is likely to result in higher batting averages for contact-type hitters.
Update
In July 2003, the crack team of Professor Dan Russell of Kettering University, Professor Lloyd Smith of Washington State University, and I did a series of measurements on several wood bats provided by Rawlings, to whom we express our thanks and gratitude. The measurements utilized the bat testing facility at the Sports Science Laboratory at Washington State (http://www.mme.wsu.edu/~ssl), of which Lloyd is the founder and director. The test consists of firing a baseball from a high-speed cannon at a speed of approximately 110 mph onto a bat that is clamped at the handle to a pivoting structure. The speed of the incoming and rebounding ball are measured, and kinematic equations are used to determine the ball-bat COR.
The primary bat we used was a 34” bat with an unmodified weight of 30.5 oz. The unmodified bat was impacted a total of 6 times. Then a cavity 1” in diameter and 10” deep was drilled into the barrel of the bat, reducing the weight to 27.6 oz. This “drilled” bat was impacted a total of 6 times. Then the cavity was filled with crushed-up pieces of cork (from wine that I had enjoyed the preceding two weeks!), raising the weight to 28.6 oz. This corked bat was impacted 12 times. Then the cork was removed and the drilled bat was impacted again 5 times. Unfortunately, the bat broke at the handle on the last impact. We had intended to fill the cavity with superball material, but that part of the experiment was cut short by breaking the bat. All impacts used the same baseball and all were at the same location, 5” from the barrel end of the bat. Various checks were done to assure that the properties of the ball did not change in the course of the measurements. A summary of our results is given in Figure 2. These data demonstrate that there is no measurable trampoline effect when a wood bat is drilled or corked.
QuesTec is a digital media company known mostly for its Umpire Information System (UIS) which is
used by Major League Baseball for the purpose of providing feedback and
evaluation of Major League umpires. The QuesTec company, based out of Deer Park, New York, has been mostly involved in television replay and
graphics throughout its history. In 2001, however, the company signed a
5-year contract with Major League Baseball to use its pitch tracking
technology as a means to review the performance of home plate umpires
during baseball games. The contract has continued through the 2008 season by annual extension and topped out at 11 ballparks.
 In 2009 it was replaced by MLB's Zone Evaluation.
In 2009 it was replaced by MLB's Zone Evaluation.
Major League Baseball has contracted QuesTec to install, operate, and maintain the UIS in support of MLB's previously announced strike zone initiatives. The UIS uses QuesTec's proprietary measurement technology that analyzes video from cameras mounted in the rafters of each ballpark to precisely locate the ball throughout the pitch corridor. This information is then used to measure the speed, placement, and curvature of the pitch along its entire path. The UIS tracking system is a fully automated process that does not require changes to the ball, the field of play, or any other aspect of the game. Additional cameras are mounted at the field level to measure the strike zone for each individual batter, for each individual pitch, for each at bat. This information is compiled on a CD ROM disk and given to the home plate umpire immediately following each game.
The UIS uses QuesTec's proprietary measurement technology. Quite different than "video insertion" technology that simply adds graphics to the broadcast video, QuesTec technology actually measures information about interesting events during the game that would not be available any other way. This technology is so innovative it appeared in a Scientific American article in September of 2000. The ball tracking component uses cameras mounted in the stands off the first and third base lines to follow the ball as it leaves the pitcher's hand until it crosses the plate. Along the way, multiple track points are measured to precisely locate the ball in space and time. This information is then used to measure the speed, placement, and curvature of the pitch along its entire path. The entire process is fully automatic including detection of the start of the pitch, tracking of the ball, location computations, and identification of non-baseball objects such as birds or wind swept debris moving through the field of view. No changes are made to the ball, the field of play, or any other aspect of the game, to work with QuesTec technology. The tracking technology was originally developed for the US military and the company has adapted it to sports applications.
Major League baseball replaced the QuesTec system with Zone Evaluation in all ballparks during the 2009 season, with triple the data collection. The system records the ball's position in flight more than 20 times before it reaches the plate. After each umpire has a plate assignment, the system generates a disk that provides an evaluation of accuracy and illustrates any inconsistencies with the strike zone. Zone Evaluation operated successfully in 99.8 percent of the 2,430 games played during the 2009 season, according to MLB.
But, umpires have pointed out, the accuracy of the system suffers once a pitch enters the strike zone — because the zone hovers above the five-sided plate as more of a three-dimensional prism, not the rectangle that television viewers see. They have maintained that although QuesTec (like Zone Evaluation) collects data in three dimensions, a hitter’s position in the batter’s box or distractions like bat movement can cloud the information, making it unfit for evaluative decisions about umpires.
Background::J.D. Drew hit a monster home run during the 1997 season, but it hit a tree in flight (while still 85' off the ground) so the length of the homer could not be determined. After reading an article in the newspaper about this problem, including some estimates by the coaches and a request for some help ("Now there's a science problem for you," FSU coach Mike Martin said. "We ought to get one of our science professors over to calculate how far that might have gone."), I stopped by practice to find out more and see if I could help. The two letters to Coach Martin included below were the result.
The first letter gives relevant data obtained from a conversation with the coach and a first estimate, while the second letter gives a summary of my numerical findings. The numerical model in my program is based on the equations and tabulated drag coefficients in ``The Physics of Baseball'' by Robert K. Adair.
First Letter
Coach Mike Martin
Moore Athletic Center
FSU Campus 4043
Dated: February 5, 1997
Dear Coach Martin:
I thought it would be useful to summarize my conclusions about the length of the home run J.D. Drew hit last weekend, stating the facts as I know them at this time and an estimate of the distance the ball would have traveled. As I told you on the field yesterday, a conservative estimate puts the home run at about 500'. It could be longer, but I need to do some calculations as described below to estimate the effect of a following wind and a lower trajectory.
The one number that I consider reliable is the distance to the fence where the ball went out. You told me 325', and this is consistent with what I would expect for a point about 2/3 of the way between the line (307') and the light tower (339'). I paced off the distance from the wall to under the top of the tree as being about 100'. It will be convenient to use 430' for the total distance to the tree. I agree with the estimate that the ball hit the tree about 80' to 90' up. Improving the accuracy of these numbers would help some, but the answer will always be uncertain.
My estimate of where the ball would have landed is obtained from a graph in ``The Physics of Baseball'' by Robert Adair. His calculations have some absolute uncertainty (that is, the speed required for a particular trajectory might be wrong), but the key thing we need is the shape -- the curvature -- of the trajectory on its downward flight. This is probably quite good for our purposes, but his graph does assume the ball was hit at the optimum angle of 35 degrees.
We can use Adair's graph to bracket where the ball would land based on the numbers above. An upper limit would be if the ball was 90' high at 435' from the plate; it would land about 510' away. This ball would have "left" the bat at 130 mph. A lower limit would be if the ball was 80' high at 425' away; it would land about 490' out, having left the bat at about 125 mph. Either would have been in level flight and about 130' high when going over the fence.
Based on comments in the paper and from a maintenance man I talked to, it seems likely that the ball was hit on a lower trajectory and therefore much harder, which is reasonable since an aluminum bat was used. The weather forecast suggests there might have been as much as a 10 mph following breeze, which also helps the ball carry. These would, I believe, increase the distance to the final landing point, but to quantify this I will have to put together a program to repeat the calculations Adair did. I will let you know what I learn. In the meantime, I think it is safe to say that the ball would have traveled at least 500', and possibly more.
By the way, descriptions of Reggie Griggs' home run suggest it was close to 500' if it did hit in that old oak tree. If it was hit higher in the air than J.D.'s ball, that would suggest a flatter and longer trajectory for Drew's homerun than this initial estimate.
Thanks for taking the time to talk to me during practice.
Sincerely, ...
Second Letter
Coach Mike Martin
Moore Athletic Center
FSU Campus 4043
Dated: February 7, 1997
Dear Coach Martin:
As I wrote in my previous letter concerning an estimate of the actual length of J.D. Drew's home run last weekend against UNC-Asheville, if the ball was hit on a lower trajectory -- that is, more of a line drive than a fly ball -- it would travel further than the minimum distance of 500' I estimated from a graph in ``The Physics of Baseball'' by Robert Adair. In order to say more, it was necessary to assemble a computer program that did the same calculation shown in Adair's book. That has now been done, and my results appear to be the same within the accuracy of the graphs included in the book. As a reminder, relative effects (like the downward trajectory of a hit ball) are the most reliable predictions of such a model.
I attach a graph that shows a variety of trajectories that (except for a 400' fly ball included for comparison) all go through the same point on the tree, 85' up and 430' away from home plate. The solid curve is the 500' fly ball described in the last letter. The longest shot, landing over 550' away, is possible if the ball is hit very hard, almost 10 % harder than the 500' fly ball, on a much lower trajectory. It barely gets over 100' in the air and would have been still rising as it went over the fence. The curves in-between are at an intermediate angle, one showing the effect of a following wind.
In conclusion, Drew's home run was probably in the 520' to 550' range and could have been longer. Comparison of these curves to what various witnesses saw should allow you to get a better estimate of how long it was. For example, if it never got much higher that a 400' batting practice shot that hits in the street out there, Drew's home run would have been in the 550' territory.
Give my regards to J.D.
Graph Included with Second Letter
click for full view
Both axis are in feet.
This drawing has an exaggerated vertical scale.
The legend in the upper corner (from gnuplot) will be relocated when I get a chance to clean up the drawing. The solid curve is on the optimal 35 degree trajectory, launched at 125 mph. The longest ball was hit at 136 mph at 25 degrees. They were in flight for about 6 seconds, as the half-second marks show.
Additional Remarks
It should be obvious that I did not include any technical remarks in my letter to Coach Martin, for obvious reasons. You may note that I did document my assumptions about the data upon which the calculational estimates are based, but not much else.